General Properties of Gases (States of Matter)

Properties of Different States.png

Mass is not density (gas has a fixed mass, same number of particles)

Kinetic Molecular Theory of Gases (KMT)

Laws that govern gas phase
Four postulates

Gas particles are in constant random motion (due to weak IMFs, shown with arrows) and exert pressure (due to motion of particles, imparts a force as it moves through space)

$Diagram not to scale (volume is 1000x greater than if solid or liquid). Gas particles constantly move (have kinetic energy) around and impart a force (pressure, $KE={\frac {1} {2}}mv^2$ where v is velocity).$

Diagram not to scale (volume is 1000x greater than if solid or liquid). Gas particles constantly move (have kinetic energy) around and impart a force (pressure, $KE={\frac {1} {2}}mv^2$ where v is velocity).

Gas particles are considered to have no volume (negligible amount, doesn’t matter compared to the container); gas particles are considered to be point particles (particles that take up no space/volume)
- Different elements have different size particles, but 1 mole occupies the same amount of space (volume) since the particles are so far away that only the overall volume matters (individual particles don’t)
- One mole of any gas takes up 22.4 L at STP (standard temperature and pressure, 0ºC and 1 atm)

Draw gas particles as a point, size of particles is zero (actually false but a good approximation, similar to how electrons’ mass is negligible)

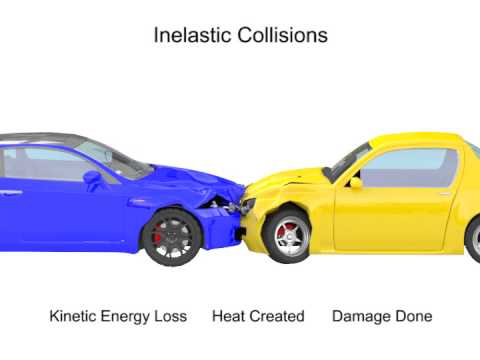
Gas particles behave elastically and have no attractive forces (IMFs)
- Elastic vs. inelastic collisions
  - Inelastic collisions do not conserve kinetic energy, less energy after collisions
  - Elastic collisions never lose energy after (same amount of energy after the collision)
- No attractive forces because the distance between other gas particles is so large that the amount of force approaches zero, too far to experience attractive forces ($F={\frac {kQ_1Q_2} {r^2}}$, r ⬆️ F ⬇️)
  - Condense (put pressure on the particles, decrease radius) to turn gas into a liquid

The kinetic energy of the gas particles is proportional to their temperature in Kelvin (K)
- Kelvin is an absolute scale of temperature (no negative values), necessary in calculations
  - ºC + 273 = K
  - K does not have a degrees symbol
  - Kinetic energy of the gas particles is not directly proportional to their temperature in ºC
- Temperature measures the average kinetic energy of the particles ($KE_{avg}={\frac {3} {2}}RT$, KE ⬆️ T ⬆️) and the speed/velocity of the particles ($KE={\frac {1} {2}}mv^2$, KE ⬆️ v ⬆️)
  - T ⬆️ KE ⬆️ v ⬆️
    - Velocity of the particle also depends on the particle’s mass (smaller particles move faster)
  - As kinetic energy increases, the entire Boltzmann distribution does not shift, the graph becomes wider (tail still hooked onto origin)
  - The total area under the curve (total number of particles) is the same, graph become wider/flattens (higher temperature) or narrower (lower temperature), different distribution
  - Higher KE means more/greater distribution possible (wider)

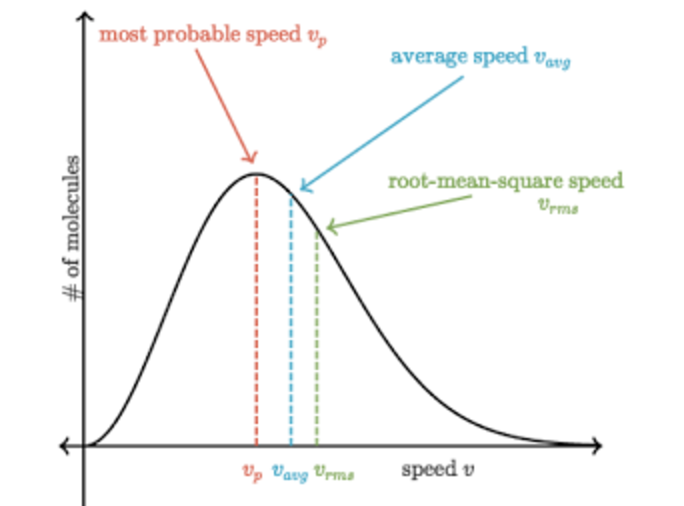
The average velocity/kinetic energy is what the thermometer measures, but particles can be slower/faster (represented by the rest of the curve).

$Boltzmann distribution - The y-axis is the number of particles. The x-axis is the velocity/kinetic energy. $T_{red} < T_{green} < T_{blue}$$

Boltzmann distribution - The y-axis is the number of particles. The x-axis is the velocity/kinetic energy. $T_{red} < T_{green} < T_{blue}$

Units of Pressure

Typically, amounts of solids are measured by mass, amounts of liquids are measured in volume, and amounts of gas are measured in pressure

<aside> 🎈 Pressure measures the frequency of gas particle collisions (each collision exerts force)

</aside>

$Pressure={\frac {Force} {area}}$
- Force of collision has 2 factors: velocity and mass